With the first Presidential Debates tonight, the election season is reaching a fever pitch. There were several places I could go with the election cycle. For example, Steve Brams’ piece on how game theory helps explain Trump's strategies. However, it seems an appropriate time to write about our Two Candidate Election Game.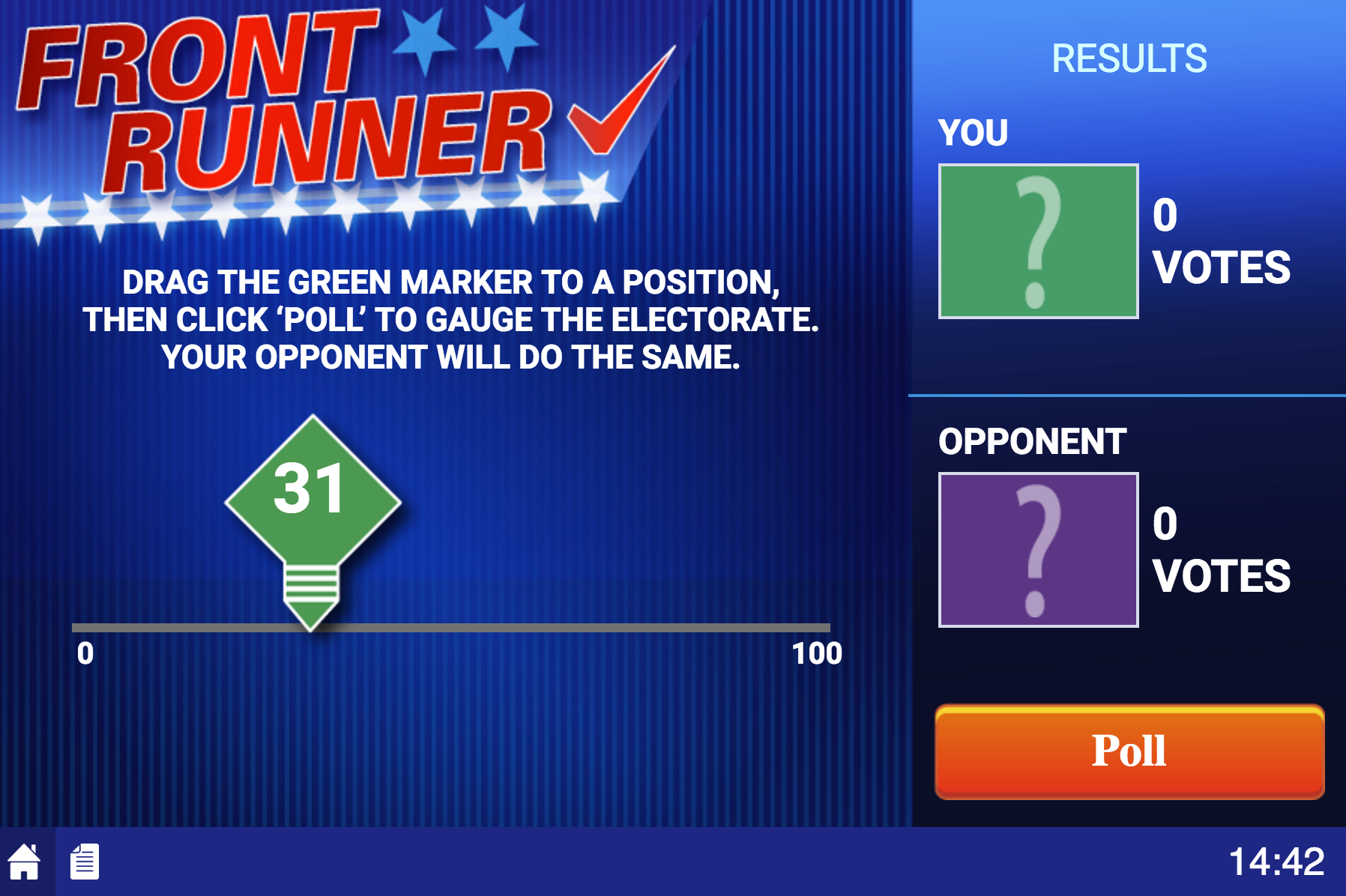 In this game students are politicians seeking to win an election. The default parameters structure the game as follows:
In this game students are politicians seeking to win an election. The default parameters structure the game as follows:
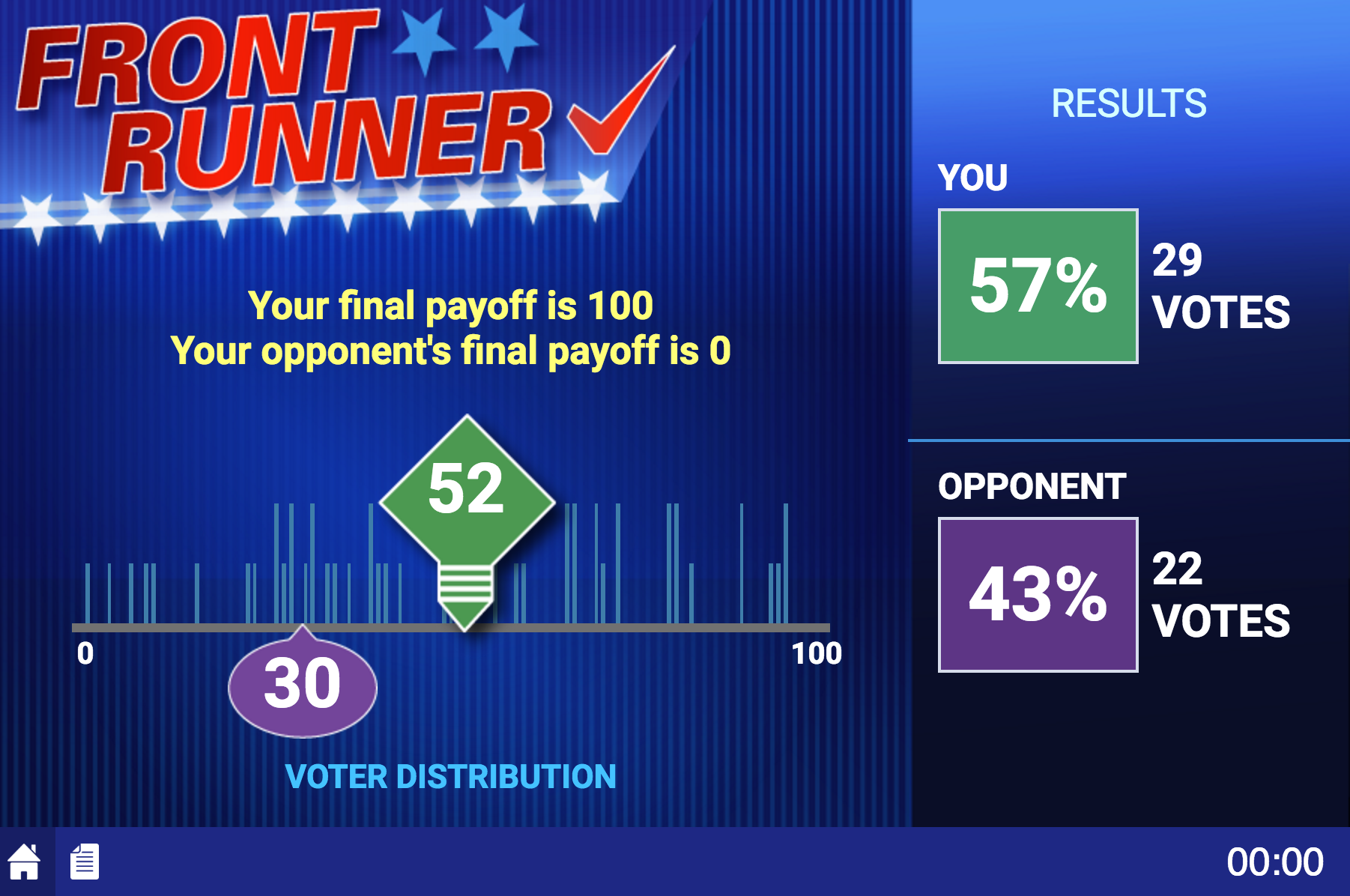
Payoff to winner: Although the default winning payment is 100 it can be manipulated to increase or decrease the stakes of the election. Payoff type “ideal point”: The default payoff is “winner take all” and even though a candidate might have some policy preferences it does not factor into their payment. On the contrary, switching to payoff type “ideal point” supposes that a politician cares about about a particular policy position and receives disutility when departing from that position. With “ideal point” the payoffs to the winner areWinning Payment + 50 - Distance from Ideal Point. The payoffs to the loser are: 0 + 50 - Distance from Ideal Point. Alter the median voter position: Make sure that the students are paying attention. Increase the number of polls prior to an election: Increasing the number of elections will help student learning. While we won't go as far as our political science friends at Stony Brook to make any predictions about this election, we can say--with a high level of confidence--that your students will really enjoy this game.
 In this game students are politicians seeking to win an election. The default parameters structure the game as follows:
In this game students are politicians seeking to win an election. The default parameters structure the game as follows:There are two candidates that make a choice about how to locate on a line that ranges from 0 to 100. An odd number of voters (in the default 51) are drawn from a uniform distribution around the median voter (default 50). Each candidate has an opportunity to “poll” twice. A poll reveals the following to the student: Given that you chose to locate at position X and your opponent chose to locate at Y the vote outcome was the following:

Students do the poll again. Then, after the polling feedback each chooses a “policy position”. With the default parameters the payoffs are based on “winner take all” where the winner receives 100 and the loser receives 0.